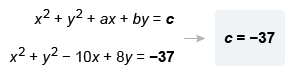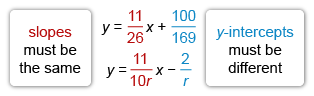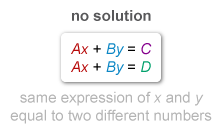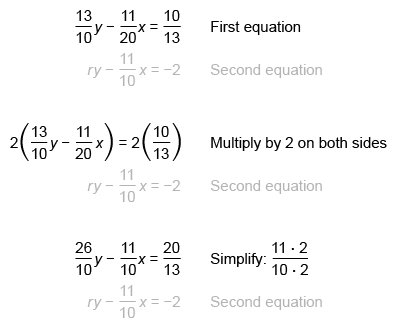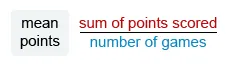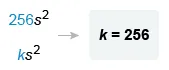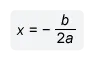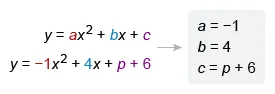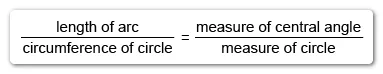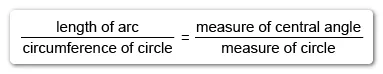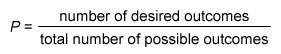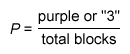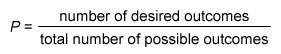Free SAT Math Prep Questions, Problems & Tests
Sharpen your skills with free SAT® math problems that match the exam, along with practice tests, video lessons, and more inside our free trial.
Try Free SAT Math Practice Problems
Other SAT prep resources end when you answer. We go further with detailed explanations and visuals that teach you the “why” behind every correct and incorrect answer. Ready to feel the difference?
Sample SAT Math Practice Test 1
Hard SAT Math Questions
SAT Math prep for the hardest problems helps you confidently tackle challenging questions. By practicing at this level, you’ll unlock the highest possible scores.
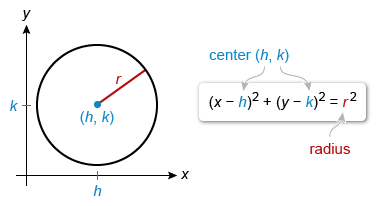
An equation of a circle in the xy-plane with radius 2 and center at (5, −4) is x2 + y2 + ax + by = c. What is the value of c ?
Submit Next Question
Hint: The equation of a circle in standard form is (x − h)2 + (y − k)2 = r 2.
Correct Answer: -37
The equation of a circle in standard form is (x − h)2 + (y − k)2 = r 2, where (h, k) is the center and r is the length of the radius.
The given circle has radius 2 and center at (5, −4), so plug in r = 2 and (h, k) = (5, −4) to get an equation of the circle in standard form. Then rewrite the equation in the form x2 + y2 + ax + by = c to identify the value of c.
| (x − h)2 + (y − k)2 = r 2 | Standard form |
| (x − 5)2 + (y − (−4))2 = 22 | Plug in (h, k) = (5, −4) and r = 2 |
| (x − 5)2 + (y + 4)2 = 4 | Simplify |
Now expand the perfect squares (x − 5)2 and (y + 4)2 and then rearrange the equation to the form x2 + y2 + ax + by = c.
| (x − 5)2 + (y + 4)2 = 4 | |
| x2 − 10x + 25 + y2 + 8y + 16 = 4 | Expand (x − 5)2 and (y + 4)2 |
| x2 + y2 − 10x + 8y + 41 = 4 | Rearrange and combine constant terms on left |
| x2 + y2 − 10x + 8y = −37 | Subtract 41 from both sides |
Compare the resulting equation to the given equation to identify c.
Things to remember:
- The equation of a circle in standard form is (x − h)2 + (y − k)2 = r 2, where (h, k) is the center and r is the length of the radius.
- To expand (a + b)2, use the identity (a + b)2 = a2 + 2ab + b2 or rewrite the expression (a + b)2 as (a + b)(a + b) and multiply.
In a warehouse, there are 7 shelves of cardboard boxes and 8 shelves of plastic boxes. Each shelf of cardboard boxes has 12 boxes that weigh 25 pounds or more and 8 boxes that weigh less than 25 pounds. Each shelf of plastic boxes has 11 boxes that weigh 25 pounds or more and 9 boxes that weigh less than 25 pounds. A box from one of these shelves will be selected at random. What is the probability of selecting a plastic box, given that the box is 25 pounds or heavier?
Submit Next Question
Hint : To find the probability of a desired event, use the following formula:
P = number of desired outcomes / total number of possible outcomes
Correct Answer: 22/43
The question asks for the probability of selecting a plastic box, given that the box is 25 pounds or heavier.
To find the probability of a desired event, use the following formula:
P = number of desired outcomes / total number of possible outcomes
The total number of possible outcomes is the total number of boxes that are 25 pounds or heavier.
The number of desired outcomes is the number of those boxes that are plastic.
The total boxes that weigh 25 pounds or more is the sum of the numbers of plastic boxes and cardboard boxes that weigh 25 pounds or more.
It is given that there are 8 shelves with 11 plastic boxes on each shelf that weigh 25 pounds or more and that there are 7 shelves with 12 cardboard boxes on each shelf that weigh 25 pounds or more.
Multiply each number of shelves by the number of boxes on each shelf to see that there are 8 ∙ 11 = 88 plastic boxes and there are 7 ∙ 12 = 84 cardboard boxes that weigh 25 pounds or more.
P = 88 / 88 + 84
Now simplify the probability.
| Add in denominator | |
| Simplify 88 / 172 = 4 . 22 / 4 . 43 |
The probability of selecting a plastic box, given that the box is 25 pounds or heavier, is 22 / 43.
Things to remember:
To find the probability of a desired event, use the following formula:
P = number of desired outcomes / total number of possible outcomes
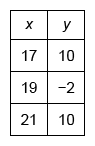
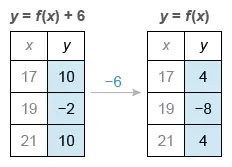
The table shows three values of x and their corresponding values of y, where y = f(x) + 6 and f is a quadratic function. What is the y-coordinate of the y-intercept of the graph of y = f(x) in the xy-plane?
Submit Next Question
Hint: For any function f(x), the graph of y = f(x − h) + k is the graph of y = f(x) transformed by a horizontal shift of h units and a vertical shift of k units.
The equation of a quadratic function in vertex form is y = a(x − h)2 + k, where (h, k) is the vertex of the parabola and a is the scale factor.
Correct Answer: 1075
The equation y = f(x) + 6 is in terms of f(x), so it is the result of a transformation to the function f(x). The constant 6 is added to the output f(x), so it represents a vertical shift 6 units up.
Each row of the table represents an (x, y) point on the graph of y = f(x) + 6. The question asks for the y-intercept of f(x), so apply the transformation to the given table to get a table of values for y = f(x).
Every point on the graph of y = f(x) + 6 is 6 units above a point on the graph of y = f(x), so subtract 6 from each y-value to get a table that represents points on the graph of y = f(x).
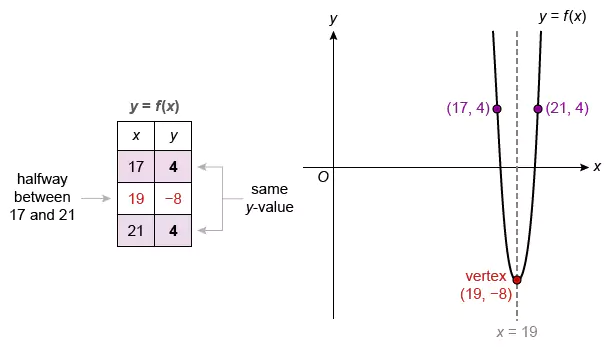
It is given that f(x) is a quadratic function, so its graph is a parabola. A vertical axis of symmetry passes through the vertex and is located halfway between any two points on a parabola that have the same y-value.
Notice that two points from the table have the same y-value (4). Therefore, the point with an x-value halfway between 17 and 21 is the vertex (h, k). This point is in the table (19, −8).
Plug (h, k) = (19, −8) into the vertex form equation y = a(x − h)2 + k to see that an equation for the graph of f(x) is y = a(x − 19)2 − 8. Plug in a different point from the table to solve for a. Choose (x, y) = (17, 4).
| y = a(x − 19)2 − 8 | |
| 4 = a(17 − 19)2 − 8 | Plug in x = 17 and y = 4 |
| 4 = a(−2)2 − 8 | Subtract inside parentheses |
| 4 = 4a − 8 | Apply the exponent |
| 12 = 4a | Add 8 to both sides |
| 3 = a | Divide by 4 on both sides |
Plug a = 3 into y = a(x − 19)2 − 8 to see that an equation for f(x) is y = 3(x − 19)2 − 8. To find the y-coordinate of the y-intercept, plug in x = 0 and solve for y.
| y = 3(x − 19)2 − 8 | |
| y= 3(0 − 19)2 − 8 | Plug in x = 0 |
| y = 3(−19)2 − 8 | Subtract inside parentheses |
| y = 3(361) − 8 | Apply the exponent |
| y = 1,075 | Simplify |
Note: It is also possible to use the given table of values to see that the equation of the transformed function is y = 3(x − 19)2 − 2 and has y-intercept 1,081. Then subtract 6 to get the y-coordinate of the y-intercept of f(x).
Things to remember:
- For any function f(x), the graph of y = f(x − h) + k is the graph of y = f(x) transformed by a horizontal shift of h units and a vertical shift of k units.
- A vertical axis of symmetry passes through the vertex (h, k) of a parabola and is located halfway between any two points on a parabola that have the same y-value.
- The equation of a quadratic function in vertex form is y = a(x − h)2 + k, where (h, k) is the vertex of the parabola and a is the scale factor.
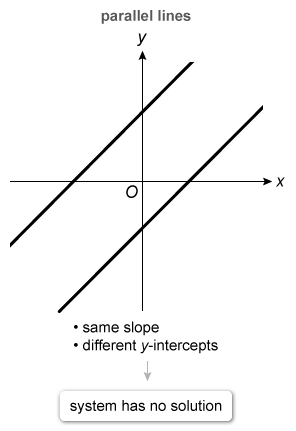
In the system of equations shown, r is a constant. If the system has no solution, what is the value of r ?
Submit Next Question
Hint : A system of linear equations has no solution if the same expression of x and y is equal to two different numbers.
Correct Answer: 26/10
It is given that the system has no solution, so its graph must consist of lines that do not intersect. Therefore, the lines must be parallel. Parallel lines have the same slope but different y-intercepts.
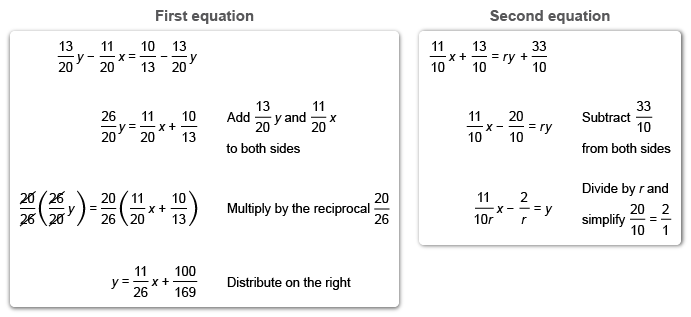
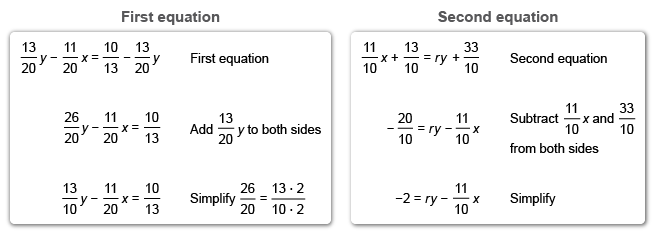
Rewrite the given system of linear equations in slope-intercept form y = mx + b, where m is the slope and b is the y-intercept. Isolate the y-term in the first equation.
Rewrite the second equation to align like terms, then compare the two equations to identify the slopes and y-intercepts.
Set the slopes equal and solve for r to find the value of r that makes the slopes the same.
| 11 / 26 = 11 / 10r | Set slopes equal |
| ( 11 / 26 )r = ( 11 / 10r )r | Multiply both sides by r |
| 26 / 11 ( 11 / 26 )r = ( 11 / 10 ) 26 / 11 | Multiply both sides by 26 / 11 , the reciprocal of 11 / 26 |
| r = 26 / 10 | Simplify |
For the y-intercepts to be different and the slopes to be the same, the value of r must be 26 / 10 .
Note: It is possible to plug r = 26 / 10 into the second equation to verify that the y-intercepts are different (graph).
Things to remember:
- A system of linear equations has no solution if its graph consists of two parallel lines because parallel lines never intersect.
- Parallel lines have the same slope but different y-intercepts.
Alternate Method :
A system has no solution if the same expression of x and y is equal to two different numbers.
Rewrite the given system of linear equations in standard form so that the constant terms are isolated.
Now each equation is written in standard form. Notice that is a multiple of . Multiply the first equation by 2 so that the x-terms are the same () and .
The x-terms are the same ( and ) and the constant terms are different (), so identify the value of r that makes the y-terms the same.
Therefore, the value of r must be for the system to have no solution.
Things to remember:
A system has no solution if the same expression of x and y is equal to two different numbers.
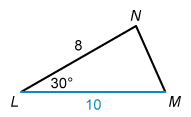
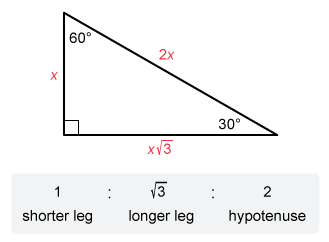
In triangle LMN, the measure of angle L is 30°, the length of LM is 10 units and the length of LN is 8 units. What is the area, in square units, of triangle LMN?
Submit Unlock Questions
Hint : The height of a triangle is the perpendicular distance from the base of the triangle to the opposite vertex.
Correct Answer: 20
The formula for the area of a triangle is , where b is the length of the base and h is the height.
Draw triangle LMN and label it with the given information. Let LM with a given length of 10 be the base of triangle and notice the height of the triangle is unknown.
The height of a triangle is the perpendicular distance from the base of the triangle to the opposite vertex.
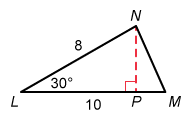
Draw a line segment from vertex N down and perpendicular to base LM . Label the new point of intersection P. The length of NP is the height of triangle LMN.
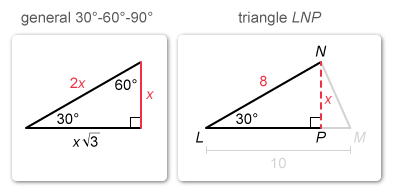
Triangle LNP is a right triangle with a given angle of 30°, so it is a 30°-60°-90° triangle.
In a 30°-60°-90° triangle, the hypotenuse is twice the length of the shorter leg. Compare the side lengths of the general triangle to triangle LNP to see that hypotenuse LN must be twice the height NP.
The length of the hypotenuse is 8, so set 2x equal to 8 and divide by 2 to find the length of the shorter leg x.
| 2x = 8 | Length of hypotenuse of special right triangle |
| x = 4 | Divide by 2 on both sides |
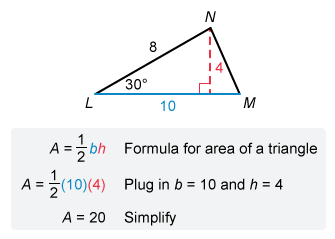
The length of the shorter leg is 4, so the height of triangle LMN is 4. Plug the length of the base (10) and the height (4) of into the formula for the area of a triangle and simplify.
The area of triangle LMN, in square units, is 20.
Things to remember:
-
The formula for the area of a triangle is , where b is the length of the base and h is the height.
- A 30°-60°-90° triangle has the following ratio of side lengths:
- The height of a triangle is the perpendicular distance from the base of the triangle to the opposite vertex.
Sample SAT Math Practice Test 2
Realistic SAT Math Questions
Quiz yourself on every question type—algebra, problem-solving and data analysis, geometry and trigonometry, and advanced math.
If a − b = 3 and a + b = 4, what is the value of (a − b)(a2 − b2) ?
Submit Next Question
Hint: Factor the expression (a2 − b2) as a difference of two squares.
Correct Answer: 36
Notice that the given expression contains a difference of two squares (a2 − b2) and a factor with a given value (a − b).
A difference of two squares of the form x2 − y2 can be factored as (x + y)(x − y).
difference of two squares
x2 − y2 = (x + y)(x − y)
The difference of two squares a2 − b2 in the given expression can be factored as (a + b)(a − b), and both factors have given values. To evaluate (a − b)(a2 − b2), first factor a2 − b2 as a difference of two squares.
| (a − b)(a2 − b2) | Given expression |
| (a − b)(a + b)(a − b) | Factor a2 − b2 |
Now plug in the given values for each of the factors (a − b) = 3 and (a + b) = 4.
| (a − b)(a + b)(a − b) | |
| (3)(4)(3) | Substitute (a − b) = 3 and (a + b) = 4 |
| 36 | Multiply |
The value of (a − b)(a2 − b2) is 36.
Things to remember:
-
An expression of the form x2 − y2 is a difference of two squares that can be factored as (x + y)(x − y).
difference of two squares
x2 − y2 = (x + y)(x − y)
- If the value for an expression is given, it may be possible to rewrite a desired expression in terms of the given expression and plug in known values.
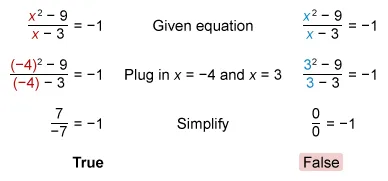
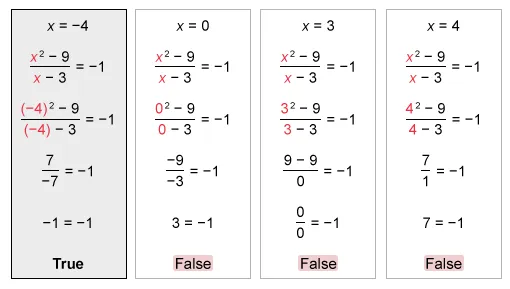
x2 − 9 / x − 3 = −1
What is the solution set of the equation above?
- {−4}
- {0}
- {3}
- {3, 4}
Hint : To solve a rational equation, first multiply both sides by the least common denominator (LCD) to clear the fractions.
The given equation x2 − 9 / x − 3 = −1 is a rational equation because it contains a variable in a denominator. Solutions to a radical equation may be valid or extraneous, so solve for x and then check for extraneous solutions.
The only denominator in x2 − 9 / x − 3 = −1 is x − 3, so multiply both sides of the equation by x − 3 to clear the fraction.
| x2 − 9 / x − 3 = −1 | Given equation |
| (x2 − 9)(x − 3) / x − 3 = −1(x − 3) | Multiply both sides by (x − 3) |
| (x2 − 9)(x − 3) / x − 3 = −1(x − 3) | Cancel factors to clear fraction |
| x2 − 9 = −1(x − 3) | Simplify |
To solve the resulting quadratic equation, rewrite it in standard form ax2 + bx + c = 0 and then factor.
| x2 − 9 = −1(x − 3) | |
| x2 − 9 = −x + 3 | Distribute −1 to (x − 3) |
| x2 + x − 12 = 0 | Add x and subtract 3 on both sides |
| (x + 4)(x −3) = 0 | Factor |
The expression (x + 4)(x − 3) is equal to 0 when either of its factors equals 0. Set each factor equal to 0 to solve for x.
| x + 4 = 0 | Set each factor equal to 0 | x − 3 = 0 |
| x = −4 | Solve for x | x = 3 |
Plug x = −4 and x = 3 into the given equation and simplify to check if they are valid or extraneous.
The solution x = −4 results in a true statement, so −4 is a valid solution.
The solution x = 3 results in a false statement, so 3 is an extraneous solution.
Therefore, the solution set of the given equation is {−4}.
Elimination strategy: It is possible to identify values of x where the given equation is undefined to eliminate Choices C and D.
(Choice B) 0 is not in the solution set, because 0 does not satisfy the given equation (see alternate method).
(Choice C) 3 is an extraneous solution, because the left side of the given equation x2 − 9 / x − 3 = −1 simplifies to the undefined fraction 0 / 0 when x = 3. Therefore, 3 is not in the solution set.
(Choice D) 3 and 4 may result from a combination of a sign error and the error described in Choice C.
Things to remember:
-
An equation that contains a variable in a denominator is a rational equation. To solve a rational equation, multiply both sides by the least common denominator (LCD) to clear the fractions.
-
Solutions to a radical equation may be valid or extraneous, so solve the equation and then plug the results into the given equation to check for extraneous solutions.
-
A rational equation is undefined when any variable denominator is equal to zero.
Alternate Method :
It is also possible to plug each possible solution from the choices into the given equation to determine the solution set. The choices include −4, 0, 3, and 4 as possible solutions.
Plug x = −4, x = 0, x = 3, and x = 4 into x2 − 9 / x − 3 = −1 to see which simplifies to a true statement.
Of the values from the choices, only −4 simplifies the equation to a true statement. Therefore, the solution set of the given equation is {−4}.
Note: When x = 3, the left side of the given equation x2 − 9 / x − 3 = −1 simplifies to the undefined fraction 0 / 0 .
Things to remember:
Any value that simplifies an equation to a true statement is a solution to the equation.
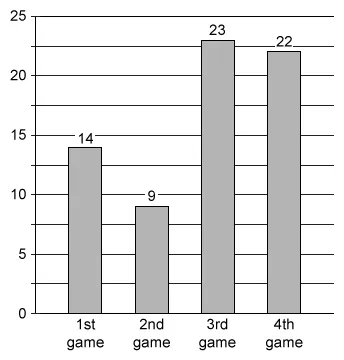
The bar graph shows the number of points scored by a football team in each of four games. For these four games, how much greater is the median number of points scored than the mean number of points scored?
Submit Next Question
Hint: The mean of a data set is the sum of the values divided by the number of values.
The median is the middle of a data set when all values are ordered numerically.
Correct Answer: 1
To find how much greater the median number of points is than the mean number of points, calculate each measure separately and then subtract the mean from the median.
First identify from the given bar graph that the the numbers of points scored in the 4 games were 14, 9, 23, and 22.
Mean score
The mean of a data set is the sum of the values divided by the number of values.
The sum of the values is the sum of the numbers of points scored and the number of values is the number of games.
Plug the numbers of points (14, 9, 23, and 22) and the number of games (4) into the expression for the mean and simplify to calculate the mean score.
| Mean number of points | |
| Plug in values | |
| Add in numerator | |
| Divide |
The mean number of points is 17.
Median score
The median is the middle of a data set when all values are ordered numerically. For a data set with an even number of values, the median is the average of the middle two.
The numbers of points scored are 14, 9, 23, and 22. Order these values numerically and then calculate the average of the middle two to find the median number of points scored.
The median number of points is 18.
The mean is 17 points and the median is 18 points. Subtract 18 − 17 = 1 to see that the the median is 1 point greater than the mean.
Things to remember:
- The mean of a data set is the sum of the values divided by the number of values.
- The median is the middle of a data set when all values are ordered numerically. For a data set with an even number of values, the median is the average of the middle two.
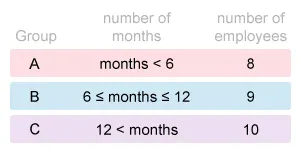
A small company classifies its employees according to how long they have been employed. Eight employees have worked at the company for less than 6 months and are classified in Group A. Nine employees have worked at the company for at least 6 months but no more than 12 months and are classified in Group B. Ten employees have worked at the company for more than 12 months and are classified in Group C. Which of the following could be the median amount of time, in months, that these 27 employees have worked at the company?
- 5.5
- 7
- 12.5
- 16
Hint : The median of a data set is the middle of the set when all values are ordered numerically.
The median of a data set is the middle of the set when all values are ordered numerically. When the number of values is odd, the median is the middle value.
The number of months each employee has worked at the company is unknown, but the range of months for each group is given. First consider the groups from least to greatest according to the ranges of months worked.
It is given that that there are 27 employees, so the median number of months must be the number worked by the 14th employee (proof).
Starting with the first range, add the number of employees in each successive group to find the group that contains the 14th employee and identify the range of months that employee has worked.
The 14th employee was in Group B, so the 14th employee worked at least 6 months and no more than 12 months.
Of the choices, only Choice B falls within this range. Therefore, the median number of months could be 7.
(Choices A, C, and D) These choices are not possible numbers of months because they do not fall within the range worked by the 14th employee (at least 6 months and no more than 12 months).
Things to remember:
The median of a data set is the middle of the set when it is ordered numerically. When the total number of values is odd, the median is the middle value. When it is even, the median is the average of the middle two values.
In a tall office building, an express elevator descends from a height of 300 feet to 20 feet at a constant rate of 8 feet per second. What type of function best models the relationship between the height of the descending elevator and time?
- Increasing linear
- Decreasing linear
- Increasing exponential
- Decreasing exponential
Hint : A function is linear if the same number is added to each value to get the next. A function is exponential if the same number is multiplied by each value to get the next.
A function is linear if the same number is added to each value to get the next (the difference is constant).
A function is exponential if the same number is multiplied by each value to get the next (the ratio is constant).
It is given that the elevator descends 8 feet per second, so the same number (−8) is added every second. Therefore, the function is linear.
As the number of seconds increases, the height of the elevator decreases. Therefore, the function is decreasing.
The function that best models the relationship between the height of the elevator and time is decreasing linear.
(Choice A) "Increasing linear" is incorrect because as time increases, the height of the elevator decreases.
(Choice C) "Increasing exponential" may result from the combination of errors described in Choices A and D.
(Choice D) "Decreasing exponential" is incorrect because the same number is added (−8) for each constant change in time. A relationship is exponential if the same number is multiplied for each constant change in time.
Things to remember:
A relationship between two variables is:
- linear if the same number is added to each value to get the next value (the difference between consecutive values is constant).
- exponential if the same number is multiplied by each value to get the next value (the ratio of consecutive values is constant).
Sample SAT Math Practice Test 3
SAT Math Calculator Practice
Use your calculator wisely to solve accurately while managing your time.
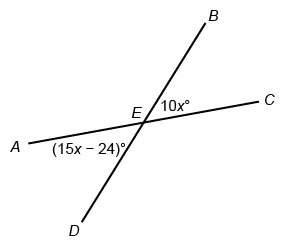
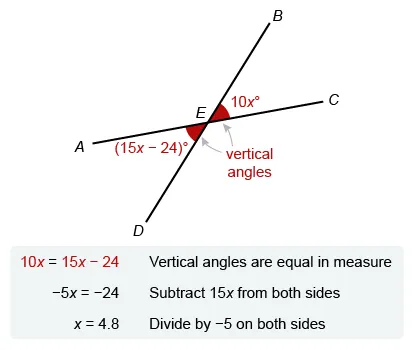
In the figure, AC and BD intersect at point E. What is the measure of angle BEC ?
- 48°
- 72°
- 82°
- 132°
Hint: When two lines intersect, they form pairs of vertical angles that are equal in measure.
When two lines intersect, they form pairs of vertical angles that are equal in measure. Therefore, vertical angles BEC and AED are equal in measure.
It is given in the figure that the measure of angle BEC is 10x° and that the measure of angle AED is (15x − 24)°. First set these measures equal and solve for x.
The value of x is 4.8. The measure of angle BEC is 10x°, so plug in x = 4.8 and multiply to see that the value of 10(4.8)° = 48°.
Note: Vertical angles BEC and AED have equal measures, so it is also possible to plug x = 4.8 into the expression for the measure of angle AED (15x − 24) to find the measure of angle BEC.
(Choice B) 72° is equal to 15x, but the measure of angle BEC is equal to 10x.
(Choice C) 82° may result from mistaking the given vertical angles to have measures that total 180° (instead of measures that are equal).
(Choice D) 132° may result from mistakenly calculating the measure of vertical angles AEB and CED (instead of the measure angle BEC).
Things to remember:
When two lines intersect, they form pairs of vertical angles that are equal in measure.
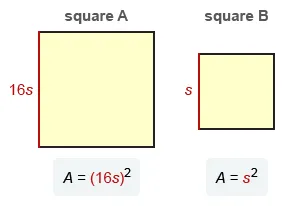
The side length of square A is 16 times the side length of square B, and the area of square A is k times the area of square B. What is the value of k?
- 4
- 16
- 32
- 256
Hint : The formula for the area of a square is A = s2, where s is the side length of the square.
It is given that the side length of square A is 16 times the side length of square B. This means that if the side length of square B is s, then the side length of square A is 16s.
The formula for the area of a square is A = s2, where s is the side length of the square. Therefore, the area of squares A and B are:
The area of square A is (16s)2 and the area of square B is s2.
It is given that the area of square A is k times the area of square B, so (16s)2 is equivalent to ks2. Simplify (16s)2 to get an expression in terms of s2.
| (16s)2 | |
| 162s2 | Use the distributive property of exponents |
| 256s2 | Simplify |
Now compare 256s2 to ks2 to find the value of k.
(Choice A) 4 may result from mistakenly taking the square root of 16 (instead of squaring 16).
(Choice B) 16 may result from mistakenly applying the exponent 2 to only s instead of both 16 and s. It is necessary to use the distributive property of exponents.
(Choice C) 32 may result from mistakenly multiplying 16 by 2 (instead of squaring 16).
Things to remember:
The formula for the area of a square is A = s2, where s is the side length of the square.
Alternate Method :
It is given that the side length of square A is 16 times the side length of square B, so if the side length of square B is s, then the side length of square A is 16s.
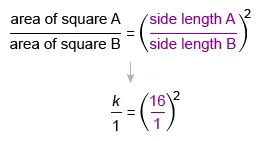
Therefore, the ratio of the side length of square A to the side length of square B is .
The ratio of the areas of any two squares is equal to the square of the ratio of the side lengths.
It is given that the area of square A is k times the area of square B, so the ratio of the area of square A to the area of square B is . Plug the ratio of the areas and the ratio of the side lengths into the proportion.
Now simplify the proportion to find the value of k.
| = ()2 | |
| Apply the exponent | |
| k = 256 | Simplify |
Things to remember:
The ratio of the areas of similar polygons is equal to the ratio of the squares of corresponding side lengths.
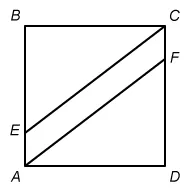
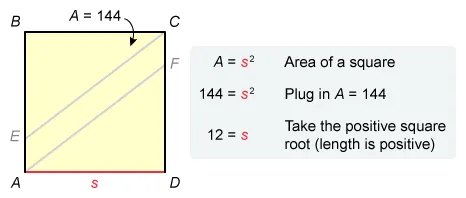
In the figure shown above, ABCD is a square and AECF is a parallelogram. The area of ABCD is 144 square feet (ft2) and the area of AECF is 36 ft2. What is the length, in feet, of line segment FD ?
- 3
- 9
- 12
- 15
Hint: Segments FD and AD are the sides of triangle AFD. Segment AD is also a side of square ABCD.
Notice that FD and AD are the legs of right triangle AFD, and that segment AD is also a side of square ABCD. Use the given area of square ABCD to find the length of AD .
The area of parallelogram AECF is also given. Use the given areas to find the area of triangle AFD, and then use the area of the triangle to find the length of FD.
length of AD
The formula for the area of a square is A = s2, where s is the side length. It is given that the area of square ABCD is 144 ft2, so plug A = 144 into the formula and solve for s.
Square ABCD has side length s = 12, so the length of AD is 12 ft
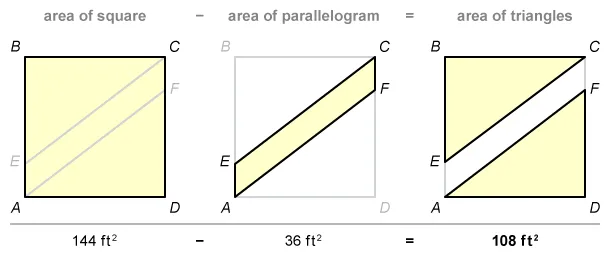
area of triangle AFD
The combined area of triangles AFD and CEB is equal to the area of square ABCD minus the area of parallelogram AECF.
The given area of square ABCD is 144 ft2 and that of parallelogram AECF is 36 ft2. Subtract to find that the combined the area of triangles ADF and CEB is 108 ft2.
Triangles AFD and CEB are congruent (proof), so they have equal areas. Therefore, the area of triangle AFD is half of the combined area.
Divide 108 by 2 to find that the area of triangle AFD is 54 ft2.
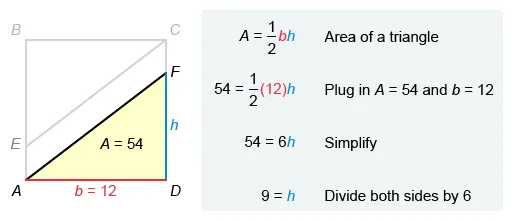
Now use the length of AD (12) and the area of triangle AFD (54) to solve for the length of FD.
The formula for the area of a triangle is , where b is the length of the base and h is the height. Let AD be the base and let FD be the height. Plug A = 54 and b = 12 into the area formula and solve for h.
The value of h is 9, so the length of FD is 9.
(Choice A) 3 is the length of the shorter side of parallelogram AECF. This may result from mistakenly finding the length of FC (instead of FD ).
(Choice C) 12 is the side length of square ABCD. This may result from mistaking the length of FD to be equal to the length of AD , but FD is not a side of square ABCD.
(Choice D) 15 is the length of the hypotenuse of triangle AFD. This may result from mistakenly finding the length of AD (instead of FD ).
Things to remember:
- The formula for the area of a square is A = s2, where s is the side length.
- The formula for the area of a triangle is , where b is the length of the base and h is the height.
- If two right triangles have congruent hypotenuses and a pair of congruent legs, the triangles are congruent (hypotenuse-leg congruence theorem).
- If two triangles are congruent, they have equal areas.
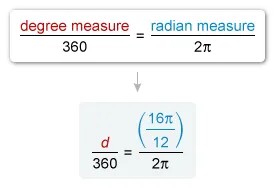
Angle P has a measure of radians. If the measure of angle Q is radians greater than the measure of angle P, what is the measure of angle Q, in degrees?
- 30
- 105
- 135
- 240
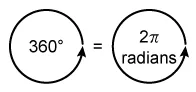
Hint : The angle measure of a complete circle is 360°, or radians.
For any angle, the ratio of its degree measure to 360° must equal the ratio of its radian measure to radians.
An angle can be measured in radians or degrees. A complete circle contains radians, or 360°.
It is given that the measure of angle Q is radians greater than that of angle P, and that the measure of angle P is radians. Add to find the radian measure of angle Q.
To add fractions, first rewrite them with a common denominator.
| Measure of angle Q | |
| Rewrite to get a common denominator: | |
| Add |
The radian measure of angle Q is radians . Let d be the equivalent degree measure and set up a proportion.
For any angle, the ratio of the degree measure to 360° must equal the ratio of the radian measure to radians. Plug in the given radian measures and then solve the proportion.
Now solve for d.
The measure of angle Q is 240 degrees.
Note: It is also possible to first find the degree measure for each angle, and then add 105 and 135 to find that the degree measure of angle Q is 240.
(Choice A) 30 may result from finding the degree measure that is radians less than (instead of greater than) the measure of angle P.
(Choice B) 105 is the degree measure that is equivalent to radians, but angle Q is radians greater than radians.
(Choice C) 135 is the degree measure that is equivalent to radians, but angle Q is radians greater than radians.
Things to remember:
The ratio of the degree measure of an angle to 360° must be equal to the ratio of the radian measure of the same angle to radians:
Alternate Method :
To convert from radians to degrees, it is also possible to multiply by a conversion factor that cancels the old unit and replaces it with the new unit.
A conversion factor is a fraction that is equivalent to 1, so it changes only the units and not the quantity. Use a fraction with the new unit in the numerator and the old unit in the denominator.
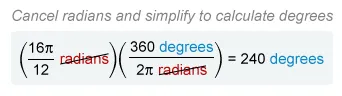
The radian measure of angle Q is (see main explanation). To convert from radians to degrees, multiply by a fraction with degrees in the numerator and the equivalent number of radians in the denominator.
The conversion is radians, so the conversion factor is . Cancel radians and simplify to find that radians is equivalent to 240 degrees.
The measure of angle Q is 240 degrees.
Note: It is possible to use an equivalent conversion 180° = radians when converting between degrees and radians.
Things to remember:
- To convert from one unit to another, multiply by a conversion factor that cancels the old unit and replaces it with the new unit.
- The conversion between degrees and radians is 360° = radians or 180° = radians.
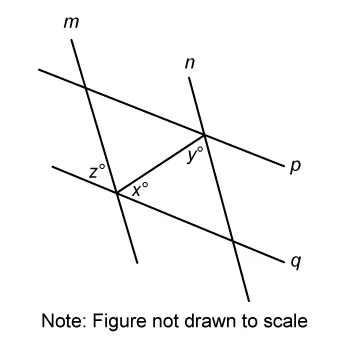
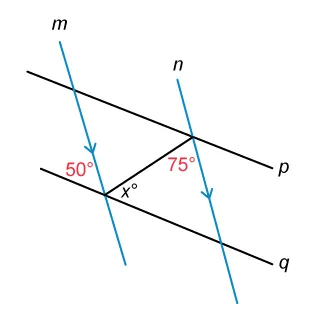
In the figure above, line m is parallel to line n. If y = 75 and z = 50, what is the value of x ?
- 50
- 55
- 60
- 65
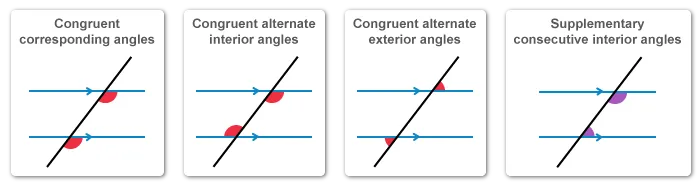
Hint : When parallel lines are intersected by a transversal, they form pairs of corresponding angles that are congruent.
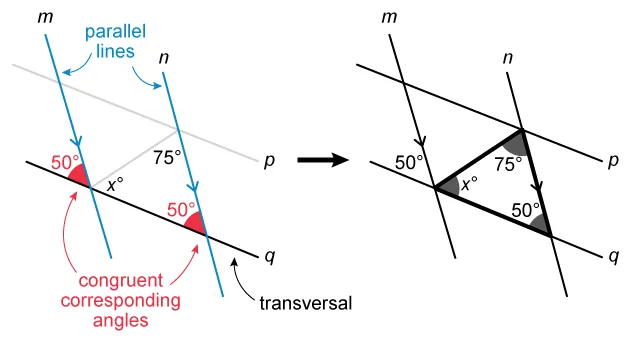
First label the figure with the given information y = 75 and z = 50, as well as m || n.
When parallel lines are intersected a transversal, the pairs of corresponding angles that they form are congruent (equal in measure).
It is given that lines m and n are parallel, so the corresponding angles below must both measure 50°. Notice that the corresponding 50° angle is also the interior angle of a triangle along with the 75° and x° angles.
The measures of the interior angles of a triangle sum to 180°, so set the sum of these interior angle measures equal to 180 and solve for x.
| x + 75 + 50 = 180 | Sum of measures of interior angles of a triangle is 180° |
| x + 125 = 180 | Simplify |
| x = 55 | Subtract 125 from both sides |
(Choice A) 50 is a result of mistaking the z° and x° angles for vertical angles and therefore equal in measure.
(Choice C) 60 is a result of the mistaken assumption that the triangle is equilateral and therefore all interior angles are equal in measure.
(Choice D) 65 is a result of a subtraction error: 180 − 125 ≠ 65.
Things to remember:
- If parallel lines are cut by a transversal, the pairs of corresponding angles that they form are equal in measure.
- The measures of the interior angles of a triangle sum to 180°.
Alternate Method :
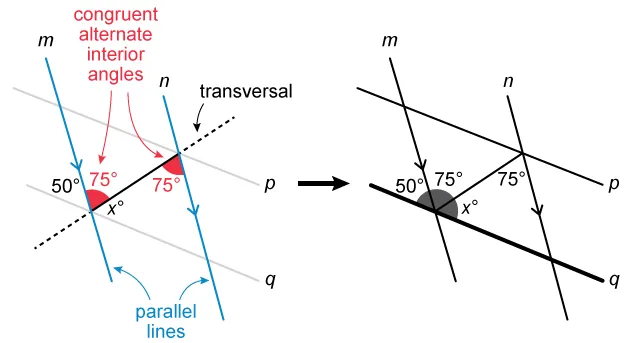
It is also possible to consider congruent alternate interior angles to find the value of x.
It is given that lines m and n are parallel, so the alternate interior angles below must both have a measure of 75°. Notice that the 50°, 75°, and x° angles combine to form a straight line (line q).
Angles that combine to form a straight line have measures that sum to 180°, so set the sum of these measures equal to 180 and solve for x.
| 50 + 75 + x = 180 | Angles that combine to form a straight line have measures that sum to 180° |
| 125 + x = 180 | Simplify |
| x = 55 | Subtract 125 from both sides |
Things to remember:
- If parallel lines are cut by a transversal, the pairs of alternate interior angles that they form are equal in measure.
- Angles that combine to form a straight line have measures that sum to 180°.
Sample SAT Math Practice Test 4
SAT Math No Calculator Practice
Many problems don’t require a calculator at all. The goal is to solve these simpler problems quickly so you can prepare for tougher questions later.
Data set P: 4, 7, 7, 10
Data set Q: 4, 7, 7, 10, 21
Data sets P and Q are shown above. Which of the following statements correctly compares the means of data set P and data set Q?
- The mean of data set P is greater than the mean of data set Q.
- The mean of data set P is less than the mean of data set Q.
- The mean of data set P is equal to the mean of data set Q.
- There is not enough information to compare the means.
Hint: Notice that data set Q is the same as data set P with an additional value of 21.
If a value is added to or removed from a data set, the mean changes unless the value added or removed is equal to the mean.
Notice that data set Q is the same as data set P with an additional value of 21, which is greater than all the other values in the data set.
Therefore, 21 must be greater than the mean of data set P. When a value greater than the mean is added to a data set, the mean of the data set increases.
Therefore, the mean of data set Q is greater than the mean of data set P and the correct statement is:
Note: Although not necessary to answer the question, it is possible to calculate and compare the mean of each data set.
Things to remember:
- If a value is added to a data set and the value is:
- less than the mean, the mean will decrease.
- greater than the mean, the mean will increase.
- If a value is removed from a data set and the value is:
- less the mean, the mean will increase.
- greater than the mean, the mean will decrease.
There are 80 employees at a company. A random sample of the company employees was selected and asked whether they intend to enroll in a new benefits option. Of those surveyed, 25% responded that they intend to enroll in the new benefits option. Based on this survey, which of the following is the best estimate of the total number of company employees who intend to enroll in the new benefits option?
- 20
- 25
- 60
- 80
Hint : A random sample is expected to represent the population it is chosen from.
A random sample is expected to represent the population it is chosen from, so the percent of surveyed employees who intend to enroll in the new option is expected to be the same as that of all company employees.
A percent of a number is equal to the percent as a decimal multiplied by that number, so the number of employees who intend to enroll is equal to the sample percent in decimal form multiplied by the total number of employees.
It is given that 25% of those surveyed intend to enroll in the new benefits option and that there are 80 total employees at the company. Divide 25 by 100 to rewrite 25% as 0.25 and then multiply by 80.
Now calculate the number of employees.
| 0.25 ∙ 80 | 25% of 80 |
| 20 | Multiply |
The best estimate of the total number of company employees who intend to enroll in the new benefits option is 20.
Note: p% is also equivalent to , so it is also possible to write 25% as and then multiply by 80 to find that the number of employees who intend to enroll is
(Choice B) 25 is the given percent of employees surveyed who intend to enroll, but the question asks for the best estimate of the total number of company employees who intend to enroll in the new benefits option.
(Choice C) 60 is the best estimate of the number of employees who do not intend to enroll in the new benefits option, but the question asks for the number who do intend to enroll.
(Choice D) 80 is the total number of employees at the company, but the question asks for the best estimate of the number of company employees who intend to enroll in the new benefits option.
Things to remember:
- A random sample is expected to represent the population it is chosen from.
- A percent of a number is equal to the percent as a fraction or decimal multiplied by that number.
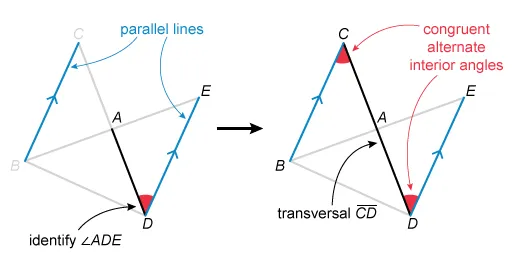
In the figure shown, BE and CD intersect at point A, and BC is parallel to DE . The measure of which of the following angles must be equal to the measure of ∠ADE ?
- ∠ABC
- ∠ACB
- ∠ADB
- ∠AED
Hint: When two parallel lines are intersected by a transversal, the pairs of alternate interior angles they form are congruent.
When two parallel lines are intersected by a transversal, the pairs of alternate interior angles they form are congruent.
It is given that BC and DE are parallel, so the intersections of transversal CD from congruent alternate interior angles ∠ADE and ∠ACB .
Therefore, the measure of ∠ADE must be equal to the measure of ∠ACB.
(Choice A) ∠ABC may result from assuming that the bottommost angles of triangles ABC and ADE are congruent.
(Choice C) ∠ADB may result from assuming that CD bisects ∠BDE , but it is only given that BC is parallel to DE.
(Choice D) ∠AED may result from assuming that triangle ADE is isosceles with base DE , but it is only given that BC is parallel to DE.
Things to remember:
When parallel lines are intersected by a transversal, the following pairs of angles are formed:
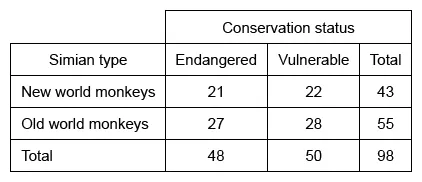
The table above shows a number of species of simians classified by type and conservation status. What is the ratio of vulnerable New World monkeys to endangered New World monkeys?
- 21 : 22
- 22 : 21
- 27 : 28
- 28 : 27
Hint : Identify the number of monkeys from the table that are in each category.
A ratio is a comparison of numbers. To find the ratio of vulnerable New World monkeys to endangered New World monkeys, identify the number of each from the table.
Identify the intersection of the Vulnerable column and the New World row.
Identify the intersection of the Endangered column and the New World row.
The choices are given in odds form, so the ratio of vulnerable New World monkeys to endangered New World monkeys is 22 : 21.
(Choice A) 21 : 22 may result from mistakenly swapping the order of the numbers in the desired ratio.
(Choice C) 27 : 28 may result from the errors described in Choices A and D.
(Choice D) 28 : 27 may result from mistakenly identifying the numbers in the Old World row (instead of the New World row).
Things to remember:
A ratio is a comparison of numbers. It is possible to express a ratio in the following forms:
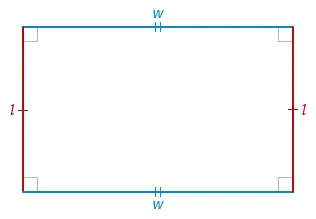
A rectangle has a length of l feet and a width of w feet. The rectangle has a perimeter of 124 feet. Which equation gives the perimeter in terms of l and w?
- 124 = l + w
- 124 = l - w
- 124 = 2l + 2w
- 124 = 2l - 2w
Hint : The perimeter of a polygon is equal to the sum of its side lengths.
The perimeter of a polygon is equal to the sum of its side lengths. A rectangle is a four-sided polygon with two pairs of opposite sides that are parallel and congruent.
The given rectangle has length l and width w, so it has two side lengths equal to l and two side lengths equal to w.
It is given that the perimeter of the rectangle is 124 feet. To determine which equation gives the perimeter, set 124 equal to the sum of the four side lengths and simplify.
| Sum of the side lengths | |
| Group like terms | |
| Combine like terms |
The equation for the perimeter in terms of l and w is 124 = 2l + 2w.
(Choice A) 124 = l + w is the sum of two side lengths, but the perimeter of a rectangle is equal to the sum of its four side lengths.
(Choice B) 124 = l − w may result from the combination of errors described in Choices A and D.
(Choice D) 124 = 2l − 2w may result from mistakenly subtracting the sum of the sides with length w from the sum of the sides with length l.
Things to remember:
- The perimeter of a polygon is equal to the sum of its side lengths.
- A rectangle is a four-sided polygon with two pairs of opposite sides that are parallel and congruent.
Sample SAT Math Practice Test 5
SAT Math Grid-In Questions
SAT Math problems include fill-in-the-blank questions, where you enter your own answer. See how you do with this custom practice test.
| y = −x2 + 4x + p |
| y = −6 |
In the system of equations shown, p is a constant. For which value of p does the system have exactly one distinct real solution?
Submit Next Question
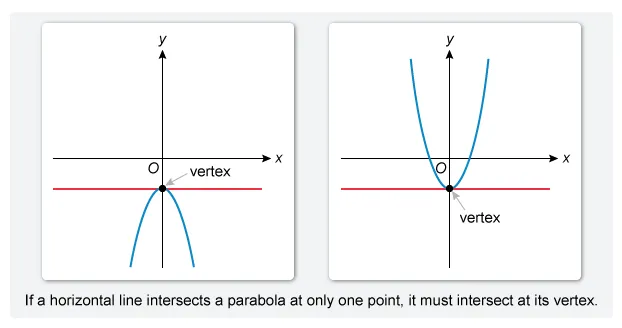
Hint: A solution to a system of equations is a point of intersection (x, y) in the xy-plane. In the given system, y = −6 represents a horizontal line and y = −x2 + 4x + p represents a parabola.
Correct Answer: -10
A solution to a system of equations is a point of intersection (x, y) in the xy-plane. In the given system, y = −6 represents a horizontal line and y = −x2 + 4x + p represents a parabola.
A parabola intersects a horizontal line at 0, 1, or 2 points. If a parabola intersects a horizontal line at a single point, then that point must be the vertex of the parabola (proof).
To find the value of p, first find the coordinates of the vertex of the parabola. Use the vertex formula for an equation in standard form y = ax2 + bx + c to find the x-coordinate of the vertex.
Compare the equation of the parabola y = −x2 + 4x + p to standard form y = ax2 + bx + c to see that a = −1 and b = 4. Plug these values into the vertex formula and simplify.
| x-coordinate of vertex | |
| Plug in a = −1 and b = 4 | |
| Simplify |
The x-coordinate of the vertex is 2. The parabola intersects the line y = −6 when the y-coordinate of the vertex is equal to −6.
Therefore, the given system of equations has exactly one distinct real solution when the vertex of the parabola is (2, −6). Plug (x, y) = (2, −6) into the equation y = −x2 + 4x + p and solve for p.
| y = −x2 + 4x + p | Given equation |
| −6 = −(2)2 + 4(2) + p | Plug in (x, y) = (2, −6) |
| −6 = −4 + 8 + p | Apply exponent and multiply |
| −6 = 4 + p | Combine constant terms |
| −10 = p | Subtract 4 from both sides |
Things to remember:
- A solution to a system of equations is a point where the graphs of the equations intersect in the xy-plane.
- If a horizontal line intersects a parabola at only one point, it must intersect at the vertex of the parabola.
- The vertex formula defines the x-coordinate of the vertex of a parabola with a quadratic equation in standard form y = ax2 + bx + c:
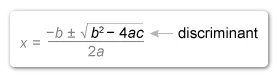
It is also possible to use the substitution method to create a single quadratic equation in terms of x, then use the discriminant to determine what value of p results in one distinct real solution.
It is given that y = −6, so substitute −6 for y in the first equation y = −x2 + 4x + p and rewrite it in standard form y = ax2 + bx + c.
| y = −x2 + 4x + p | Given first equation |
| −6 = −x2 + 4x + p | Plug in y = −6 |
| 0 = −x2 + 4x + p + 6 | Add 6 on both sides |
A quadratic equation in standard form 0 = ax2 + bx + c has exactly one solution when the discriminant b2 − 4ac is equal to 0. The discriminant is the expression under the square root in the quadratic formula.
Compare the equation 0 = −x2 + 4x + p + 6 to standard form to identify the values of a, b, and c.
Note: It is given that p is a constant, so p + 6 is also a constant.
Plug these values into the discriminant b2 − 4ac and simplify.
| b2 − 4ac | Discriminant |
| 42 − 4(−1)(p + 6) | Plug in a = −1, b = 4, and c = p + 6 |
| 16 + 4(p + 6) | Apply the exponent and simplify |
| 16 + 4p + 24 | Distribute 4 to (p + 6) |
| 40 + 4p | Combine like terms |
The discriminant is 40 + 4p, so the system has exactly one solution when 40 + 4p is equal to 0. Set the discriminant equal to 0 and solve for p.
| 40 + 4p = 0 | Set discriminant equal to 0 |
| 4p = −40 | Subtract 40 from both sides |
| p = −10 | Divide by 4 on both sides |
Things to remember:
- To find the number of solutions to a linear and quadratic system, use the substitution method to create a single equation in terms of only x.
- A quadratic equation in standard form has exactly one solution when the discriminant b2 − 4ac is equal to 0.
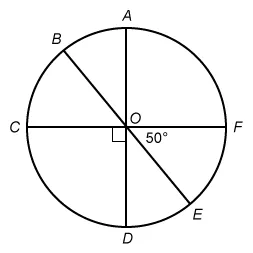
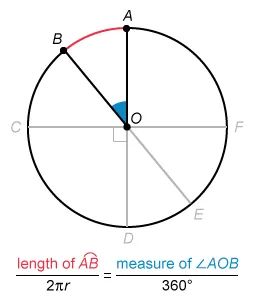
The circle shown has center O, diameters AD , BE , and CF , and the length of BO is 9. If the length of minor arc is , what is the value of ?
Submit Next Question
Hint : The length of an arc divided by the circumference of the circle must equal the measure of its intercepting central angle divided by the measure of the entire circle.
Correct Answer: 2
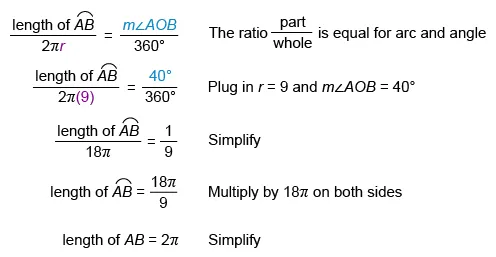
Ratios of the part to the whole are equivalent between the length of a circular arc and the measure of its intercepting central angle.
The length of an arc (part) divided by the circumference of the entire circle (whole) must equal the measure of its intercepting central angle (part) divided by the measure of the entire circle (whole), forming the following proportion:
The formula for the circumference of a circle is , where r is the length of the radius. The angle measure on the given figure is in degrees, so use 360° for the measure of a whole circle (instead of radians).
The central angle that intercepts is ∠AOB , so write the proportion as follows:
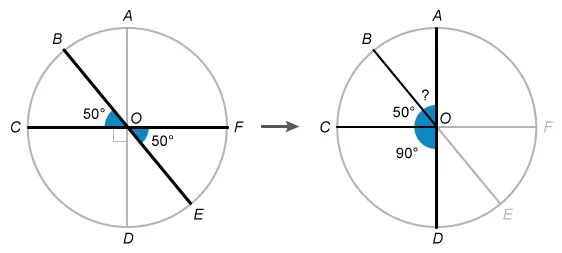
To find the measure of ∠AOB, first identify that ∠BOC and ∠EOF are a pair of vertical angles. When two lines intersect, the vertical angles formed by those lines are congruent.
Therefore, ∠BOC also has a measure of 50°. Now notice that ∠BOC forms a straight line (AD) when combined with ∠AOB and ∠COD.
Angles that combine to form a straight line have measures that sum to 180°. Set the sum of m∠AOB, 50, and 90 equal to 180, and then solve for m∠AOB.
The measure of ∠AOB is 40°. Notice that BO is a radius of the circle and has a given length of 9. Plug r = 9 and m∠AOB = 40 into the proportion, and then solve for the length of .
The length of is , so the value of k is 2.
Things to remember:
- Ratios of the part to the whole are equivalent between the area of a circular sector and the measure of its intercepting central angle. The following proportion relates circular sectors and central angles of a circle:
- The formula for the circumference of a circle is , where r is the length of the radius.
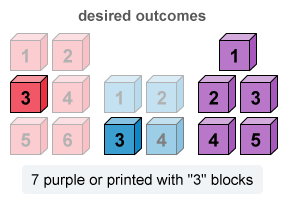
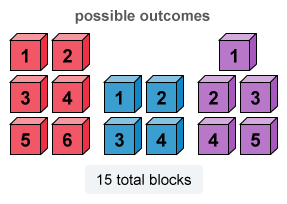
Russell's toy box contains 15 solid-colored blocks: 6 red blocks, 4 blue blocks, and 5 purple blocks. There is also a single number printed on each block. Each number from 1 through 6 is printed on a red block, each number from 1 through 4 is printed on a blue block, and each number from 1 through 5 is printed on a purple block. If Russell chooses a block at random from his toy box, what is the probability that the block will be purple or will have a 3 printed on it?
Submit Next Question
Hint: To find the probability of a desired event, use the following formula:
Correct Answer: 7/15
An item with one quality OR another quality has at least one of the qualities. To find the probability of a desired event, divide the number of desired outcomes by the total number of possible outcomes.
The number of desired outcomes is the number of blocks that are purple OR printed with a 3.
The number of possible outcomes is the total number of blocks in the toy box.
It is given that there are 15 total blocks in the toy box: 6 red blocks printed with 1 through 6, 4 blue blocks printed with 1 through 4, and 5 purple blocks printed with 1 through 5.
There are 5 purple blocks, and there are 3 blocks printed with a 3. Add 3 and 5, then subtract 1 for the 1 block that is both purple and printed with a 3 to see that there 7 blocks that are purple or printed with a 3.
Note: There is 1 block that is both purple and printed with a 3, so it is necessary to subtract 1 from the sum of the 5 purple blocks and 3 blocks printed with a 3 to avoid double-counting.
Of the 15 total blocks, there are 7 blocks that are purple or printed with a 3.
| Probability of purple or with a 3 | |
| Plug in values |
The probability that Russell chooses a block that is purple or printed with a 3 is .
Things to remember:
- To find the probability of a desired event, use the following formula:
- An item that has one quality OR another quality has at least one of the qualities (possibly both).
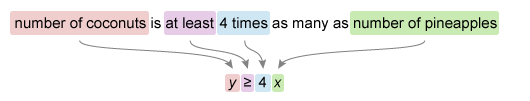
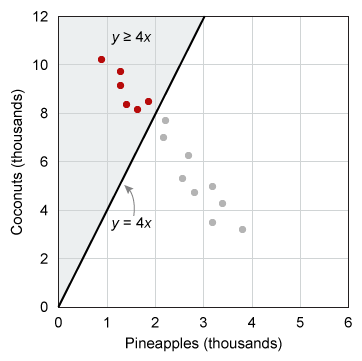
Fifteen tropical farms with equal land areas grow a combination of coconuts and pineapples. The number of coconuts and pineapples grown by each farm is shown in the scatterplot. How many farms grew at least 4 times as many coconuts as pineapples?
Submit Next Question
Hint : The question asks for the number of farms that grew at least 4 times as many coconuts as pineapples, so the number of coconuts must be greater than or equal to 4 times the number of pineapples.
Correct Answer: 6
On the given scatterplot, the number of coconuts is given on the y-axis (vertical) and the number of pineapples is given on the x-axis (horizontal).
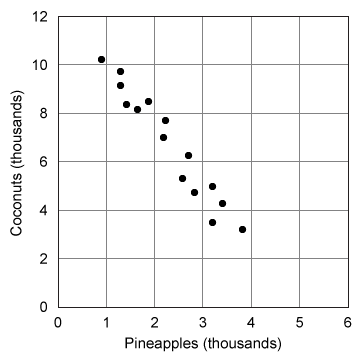
The question asks for the number of farms that grew at least 4 times as many coconuts as pineapples, so the number of coconuts (y) must be greater than or equal to (≥) 4 times the number of pineapples (x).
Therefore, the inequality y ≥ 4x represents the given relationship. To determine which points on the scatterplot satisfy the inequality, consider the graph of the boundary line y = 4x (see how).
The inequality y ≥ 4x means that any point (x, y) on or above the line y = 4x represents a farm that grew at least 4 times as many coconuts as pineapples. Count the number of points on or above the line.
There were 6 farms that grew at least 4 times as many coconuts as pineapples.
Things to remember:
To find points on a scatterplot that satisfy a relationship between two variables x and y, consider the graph of the equation or inequality on the scatterplot and identify which points satisfy the relationship.
The population of a certain town decreased by 4% from 2005 to 2015. If the population in 2015 is k times the population in 2005, what is the value of k ?
Submit
Hint : To decrease a value by p%, multiply it by ( )
Correct Answer: 0.96
The question states that the population in 2015 is k times the population in 2005.
It is also given that the population decreased by 4% from 2005 to 2015. To decrease a value by p%, multiply it by (). Therefore, the 2015 population is equal to () multiplied by the 2005 population.
Therefore, k must equal . Simplify to write the value of k as a decimal.
| Value of k | |
| Divide | |
| 0.96 | Subtract |
The value of k is 0.96.
Note: It is also possible to rewrite as a fraction and simplify the result.
Things to remember:
- To increase a value by p%, multiply it by ().
- To decrease a value by p%, multiply it by ().
Trust Our SAT Math Practice Questions
Experienced teachers, tutors, and test prep experts create our SAT Math practice questions. Each one goes through a rigorous review process to ensure accuracy, alignment with the latest test format, and coverage of essential concepts.
Get More Free SAT Math Prep Questions!
Explore More Free SAT Resources
Read more about the SAT and explore these free resources:
Students Love Our SAT Questions
Great SAT explanations which can't be found elsewhere.
Really satisfied with the quality of the math questions. They are similar to the SAT questions.
I've been using uworld for a few months now and their math section is amazing! I've improved drastically by at least 300+ points on the SAT section.
Frequently Asked Questions (FAQs)
Do these SAT math practice questions include detailed explanations?
Yes. Each SAT math problem features comprehensive explanations so you understand the reasoning behind correct and incorrect answers and avoid repeating the same mistakes on future questions.
Do these SAT math practice tests match the latest SAT format?
Yes. Our SAT math prep questions mirror the current SAT Math section format: multiple-choice and student-produced response questions that reflect the official SAT math exam structure. Students can use the full-length practice exam in our SAT Prep Course to match the 2 modules format or create custom ones focused on key topics like the sample SAT math practice tests we’ve included above.
How many SAT math practice tests are included in this product?
We provide one full-length SAT practice test, plus you can generate unlimited personalized math practice tests. To sharpen your skills, focus on weak areas, use custom tags, or revisit questions you previously missed.
How do these SAT math practice questions compare to the official SAT in difficulty?
Our SAT math problems are designed to match—or even exceed—the official SAT in difficulty, including hard questions, so you’ll be fully prepared for test day.
Do you offer additional SAT math prep resources, such as videos or tutoring?
Yes. Our SAT math prep course includes a library of video tutorials that break down challenging math concepts in simple, easy-to-follow steps. Many students say our resources are like having their own on-demand private tutor.
Can I access SAT math practice tests on multiple devices?
Yes. You can study SAT math practice questions on a computer, tablet, or smartphone, and your progress will be saved across devices for seamless SAT math prep.
How should I time myself during SAT math practice?
On the real SAT math exam, you’ll have 70 minutes for the Math section (35 minutes per module). Practice SAT math problems under these conditions or with smaller timed sets to build pacing and accuracy.
What concepts are covered on the SAT Math section?
You’ll encounter SAT math questions testing algebra, problem-solving, data analysis, geometry, trigonometry, and math in real-world contexts. Both calculator and no-calculator SAT math problems are required.
How many questions are on the SAT Math section?
There are 44 SAT math questions total, split across 2 modules. You’ll answer multiple-choice and student-produced response SAT math practice questions that mirror sample SAT math questions from official tests.
What's a good score on the SAT Math section?
SAT math test scores range from 200 to 800. Competitive colleges often look for 650–780 in Math, but your target should align with the requirements of your chosen schools. Regular SAT math practice with challenging SAT math practice problems helps achieve higher scores.