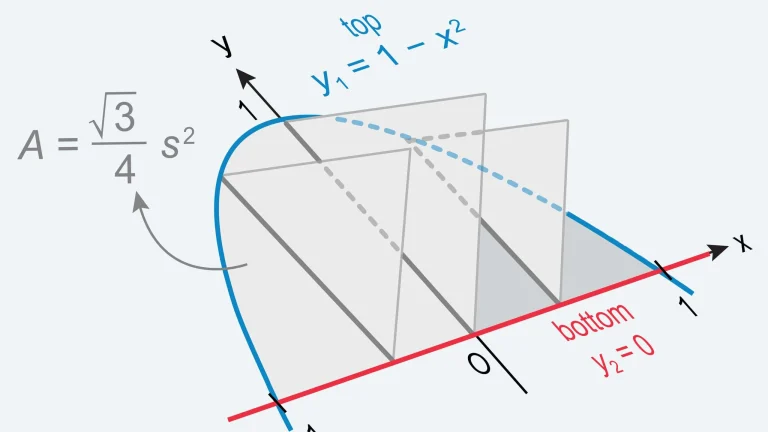
AP® Calculus BC Course And Exam Description
AP® Calculus BC is a full-year course for students wishing to pursue a career focused on mathematics or allied subjects requiring heavy math. This course is equivalent to Calculus I and II in college, and scoring well on the exam can help you skip both college courses and move on to advanced ones. Since it is one of the most extensive AP courses, preparing for it requires a detailed study plan and thorough knowledge of the course content and materials.
This guide will give you an overview of the AP Calculus BC units, topics, and key concepts taught during the course. In addition to these, we will also discuss the essential prerequisites for excelling in this course. By the end of this guide, you'll understand how to begin preparing for your upcoming Calculus BC exams and what skills to focus on.
AP Calculus BC Units, Topics, and Key Concepts
Before you learn about the AP Calculus BC course curriculum, it is vital to know about the prerequisites required to approach this course. Having these requirements set up beforehand will ease your way through the course. It also enables you to decide if you are ready to take a math-heavy course like AP Calculus BC. We've listed the prerequisites for the Calculus BC course below:
- Algebra I
- Geometry
- Algebra II
- Pre-calculus
The concepts in these courses help you build a solid foundation for reasoning with algebraic symbols and working with algebraic structures. This aspect is crucial to mastering AP Calculus BC. Make sure your high school offers courses that cover all or some of these concepts to help you prepare for the challenges of AP Calculus BC.
With the prerequisites set up, let's explore the course component. Like AP Calculus AB, the AP Calculus BC exam tests you on two components—the course content and mathematical practices. The course content combines two aspects: units and big ideas. The ten units in the AP Calculus BC curriculum revolve around three recurring themes. The College Board® labels these themes as "big ideas." In the following section, let's dive deeper into these big ideas in the next section.
AP Calculus BC’s Three Big Ideas
The AP Calculus BC units rely on three key elements or big ideas that form the basis for this course. Each of these elements is weaved into the course units as you advance through Calculus BC. Let’s take a look at the following big ideas:
- Big Idea 1: Change (CHA)
Students can understand the concept of 'change' in a variety of contexts by using derivatives to describe rates of change of one variable with respect to another or definite integrals to describe the net change in one variable over an interval of another. It is essential to understand the link between integration and differentiation as represented in the Fundamental Theorem of Calculus. This forms the essence of the first Big Idea of Change (CHA). - Big Idea 2: Limits (LIM)
Understanding essential calculus ideas, definitions, formulae, and theorems such as continuity, differentiation, and integration constitutes the second big idea of Limits (LIM).- Differentiation: Defining the derivative of a function, estimating derivatives at a point, connecting differentiability and continuity, determining derivatives of constants, sums, differences, and constant multiples and trigonometric functions. You’ll also need to learn about composite, Implicit, and inverse Functions.
- Integration: Finding the average value of a function, applying accumulation functions, finding the area between curves of functions, and finding volumes from cross-sections and revolutions. You’ll also need to study the Fundamental Theorem of Calculus, find anti-derivatives and indefinite integrals, and integrate using substitutions.
- Big Idea 3: Analysis Of Functions (FUN)
The third big idea of Analysis of Functions (FUN) enables you to understand and evaluate the behaviors of functions by relating limits to differentiation, integration, and infinite series and relating each of these concepts to the others.
As we'll discuss in the following section, these three big ideas are distributed across ten units to help students understand each concept efficiently. Both the big ideas and the course units constitute a framework for the calculus course, similar to many college courses and textbooks. Now let's discuss each of the ten course units to develop a clearer understanding of how these big ideas and the units work together to build a solid foundation for Calculus BC.
The Ten Units of AP Calculus BC and Their Topics
The units feature the study material you will learn in your AP Calculus BC course. Although the curriculum for AP Calculus BC is similar to that of the AP Calculus AB course curriculum, BC contains two additional units (Units 9 and 10), plus some extra topics in Units 6 to 8. We’ll discuss these extra units in detail, but below is a table to give you an idea:
| Unit | Additional Topics in AP Calculus BC |
|---|---|
| Unit 6 | Additional techniques of Integration |
| Unit 7 | Euler's method and logistic models with differential equations |
| Unit 8 | Arc length and distance traveled along a smooth curve |
| Unit 9 | Parametric equations, polar coordinates, and vector-valued functions |
| Unit 10 | Infinite sequences and series |
As we outline each unit, we will also look into the big ideas that spiral across the 10 course units and topics. Understanding how these topics are categorized will help you assess your target areas and know which unit and topic you need to work on during your revision. We've also included the relative weights for each unit so you can gauge the importance of these units in the AP Calculus BC exam. If you’re interested in learning more about any specific unit, click on the below unit sections.
UNIT 1: Limits And Continuity
Exam Weightage: 4–7% ｜ Class periods ~ 13–14
The first unit introduces you to the idea of change, which is the basis for studying calculus. Calculus allows us to generalize knowledge about motion to diverse problems involving change. Using the concept of limits, you’ll learn the subtle distinction between evaluating a function at a point and considering what value the function is approaching after a point in time. As you progress through this unit, you’ll learn how to determine change by justifying claims about limits and continuity through definitions, theorems, and properties.
Big Ideas Incorporated:
- Big Idea 1: Change – Can change occur in an instant?
- Big Idea 2: Limits – How does knowing the value of a limit, or that a limit does not exist, help you to make sense of interesting features of functions and their graphs?
- Big Idea 3: Analysis Of Functions – How do we close loopholes so that a conclusion about a function is always true?
In this unit, you will learn:
- How the average rate of change of a function can closely approximate the rate of change at an instant: Topic 1.1.
- What a limit is, how to express it, and how to calculate or approximate it from a table, graph, or function: Topics 1.2–1.4.
- Properties of limits and how to simplify them with algebra and trigonometry: Topics 1.5–1.7, 1.9.
- How limits of known functions can provide information about an unknown function using the squeeze theorem: Topic 1.8.
- What continuity is and how to identify when a function or graph is discontinuous at a point or over an interval: Topics 1.10–1.13.
- How to find infinite limits and limits at infinity and what information these limits can provide about a function’s asymptotes: Topics 1.14–1.15.
- How can continuity prove the existence of a function value using the intermediate value theorem: Topic 1.16.
UNIT 2: Differentiation: Definition and Fundamental Properties
Exam Weightage: 4–7% ｜ Class periods ~ 9–10
Unit 2 will expand your understanding of differentiation and its use to calculate instantaneous rates of change. You will also learn to use a graphing calculator and explore how its various operations affect tangent line slopes. This exercise will help you understand differentiation's fundamental rules and properties.
Big Ideas Incorporated:
- Big Idea 1: Change – How can a state determine the rate of change in high school graduates at a particular
level of public investment in education (in graduates per dollar) based on a model for the number of graduates as a function of the state’s education budget? - Big Idea 2: Limits – Why do mathematical properties and rules for simplifying and evaluating limits apply to differentiation?
- Big Idea 3: Analysis Of Functions – If you knew that the rate of change in high school graduates at a particular level of public investment in education (in graduates per dollar) was a positive number, what might that tell you about the number of graduates at that level of investment?
In this unit, you will learn about the following AP Calculus BC topics:
- How to find an average rate of change using a difference quotient: Topic 2.1.
- What a derivative is, its relationship to an instantaneous rate of change, and how to calculate or estimate it using the limit of a difference quotient or a table: Topics 2.2–2.3.
- How continuity and differentiability are related: Topic 2.4.
- How to calculate the derivative of different families of functions: Topics 2.5, 2.7, 2.10.
- Properties of derivatives and advanced differentiation techniques: Topics 2.6, 2.8–2.9.
UNIT 3: Differentiation: Composite, Implicit, and Inverse Functions
Exam Weightage: 4–7% ｜ Class periods ~ 8–9
Unit 3 will teach you how to differentiate composite functions using the chain rule and how to transfer that knowledge to determine the derivatives of implicit and inverse functions. The unit is based on the notion that for composite functions, y is a function of u, and u is a function of x. This is one of the most critical topics in the AP Calculus course, as excelling in the chain rule is required for success in all subsequent units.
Big Ideas Incorporated:
- Big Idea 3: Analysis Of Functions – If pressure experienced by a diver is a function of depth and depth is a function of time, how might we find the rate of change in pressure with respect to time?
In this unit, you will learn:
- More advanced differentiation techniques: Topics 3.1–3.5.
- How to differentiate a function multiple times: Topic 3.6.
UNIT 4: Contextual Applications of Differentiation
Exam Weightage: 6–9% ｜ Class periods ~ 6–7
Unit 4 begins with an understanding of average and instantaneous rates of change in motion. The unit then characterizes differentiation as a common underlying structure that can be used to understand change in various contexts. You will also explore how to use L’Hopital’s Rule to determine the limits of indeterminate forms.
Big Ideas Incorporated:
- Big Idea 1: Change – How are problems about position, velocity, and acceleration of a particle in motion over time structurally similar to problems about the volume of a rising balloon over an interval of heights, the population of London over the 14th century, or the metabolism of a dose of medicine over time?
- Big Idea 2: Limits – Since certain indeterminate forms seem to actually approach a limit, how can we determine that limit, provided it exists?
In this unit, you will learn about the following AP Calculus BC topics:
- What the derivative means in a real-world context: Topic 4.1–4.3.
- How to calculate a rate of change when two or more rates are related: Topic 4.4–4.5.
- How to approximate values of a function using a line tangent to the function at a nearby x-value: Topic 4.6.
- How to efficiently calculate difficult limits with L’Hopital’s Rule: Topic 4.7.
UNIT 5: Analytical Applications of Differentiation
Exam Weightage: 8–11% ｜ Class periods ~ 10–11
Unit 5 will concentrate on the analytical use of Differentiation to reach formal conclusions via reasoning, definitions, and theorems. You'll learn to justify their conclusions about the behavior of functions over specific intervals and the locations of extreme values or points of inflection. The unit wraps up this differentiation study by using abstract reasoning skills to justify solutions to realistic optimization problems.
Big Ideas Incorporated:
- Big Idea 3: Analysis Of Functions – How might the Mean Value Theorem be used to justify a conclusion that you were speeding at some point on a certain stretch of highway, even without knowing the exact time you were speeding? What additional information is included in a sound mathematical argument about optimization that a simple description of an equivalent answer lacks?
In this unit, you will learn about the following AP Calculus BC topics:
- How differentiability can prove the existence of a derivative value in an interval using the mean value theorem: Topic 5.1.
- What information the derivative can provide about the graph of a function like extreme values, intervals of increasing/decreasing, points of inflection, and intervals of concavity: Topics 5.2–5.9.
- How to use the derivative to optimize a quantity within a context: Topics 5.10–5.11.
- How implicit differentiation can provide information about a relation: Topic 5.12.
UNIT 6: Integration and Accumulation of Change
Exam Weightage: 17–20% ｜ Class periods ~ 15–16
One of the most important units in the AP Calculus BC course is Unit 6. Using the Fundamental Theorem of Calculus, you will understand the relationship between differentiation and integration in this unit. Integration determines the accumulation of change over an interval in the same way that Differentiation determines the instantaneous rate of change at a point. Because you'll be applying the concept of Integration to various realistic and geometric applications, this unit serves as a foundation for future units in this course.
Big Ideas Incorporated:
- Big Idea 1: Change – Given information about the population growth rate over time, how can we determine how much the population changed over a given interval of time?
- Big Idea 2: Limits – If compounding more often increases the amount in an account with a given rate of return and term, why doesn’t compounding continuously result in an infinite account balance, all other things being equal?
- Big Idea 3: Analysis Of Functions – How is integrating to find areas related to differentiating to find slopes?
In this unit, you will learn:
- What a definite integral is, its relationship with accumulation of change and area, and how to approximate the area under a curve with Riemann sums:Topics 6.1–6.5.
- Properties of definite integrals: Topics 6.6–6.7.
- What an antiderivative is and its relationship with indefinite integrals: Topic 6.8.
- Advanced integration techniques: Topics 6.9–6.14.
UNIT 7: Differential Equations
Exam Weightage: 6–9% ｜ Class periods ~ 9–10
Unit 7 will teach you how to set up and solve separable differential equations. You'll learn to convert mathematical information from one representation to another by matching equations and slope fields, rewriting verbal statements as differential equations, and sketching slope fields corresponding to their symbolic representations. You'll also learn to use Euler's method to solve problems based on real-world scenarios.
Big Ideas Incorporated:
- Big Idea 3: Analysis Of Functions – How can we derive a model for the number of computers, C, infected by a virus, given a model for how fast the computers are being infected, dC dt, at a particular time?
In this unit, you will learn:
- How to model a context with a differential equation: Topics 7.1–7.2.
- How to use a slope field to estimate solutions to differential equations: Topics 7.3–7.4.
- How to approximate a function value using Euler’s method: Topic 7.5.
- How to find a general or particular solution to a differential equation: Topics 7.6–7.7.
- How to model different growth patterns with a differential equation and how to solve it: Topics 7.8–7.9.
UNIT 8: Applications of Integration
Exam Weightage: 6–9% ｜ Class periods ~ 13–14
Unit 8 focuses on how to calculate the average value of a function, model particle motion, and net change, and calculate areas and volumes defined by graphs and functions. This unit also includes geometric integration applications. The main objective of this unit is to develop an understanding of Integration that can be applied to these and other applications.
Big Ideas Incorporated:
- Big Idea 1: Change – How is finding the number of visitors to a museum over an interval of time based on information about the rate of entry similar to finding the area of a region between a curve and the x-axis?
In this unit, you will learn:
- How to use a definite integral to find the average value of a function on an interval: Topic 8.1.
- What the definite integral means in a context: Topics 8.2–8.3.
- How to use a definite integral to find the area between curves: Topics 8.4–8.6.
- How to use a definite integral to find the volume of a solid with a cross-section of a particular geometric shape: Topics 8.7–8.8.
- How to use a definite integral to find the volume of a solid of revolution: Topics 8.9–8.12.
- How to use a definite integral to calculate the length of a curve over an interval: Topic 8.13.
UNIT 9: Parametric Equations, Polar Coordinates, and Vector-Valued Functions
Exam Weightage: 11–12% ｜ Class periods ~ 10–11
Unit 9 is exclusive to AP Calculus BC. In this unit, students will apply their knowledge of straight-line motion to solve problems involving particles moving along plane curves. They will learn to describe planar motion through parametric equations and vector-valued functions and use calculus to solve motion problems. Students will discover that polar equations are a type of parametric equation and will use calculus to analyze graphs and calculate lengths and areas.
Big Ideas Incorporated:
- Big Idea 1: Change – How can we model motion that is not constrained to a linear path?
- Big Idea 3: Analysis Of Functions – How does the chain rule help us analyze graphs defined using parametric equations or polar functions?
In this unit, you will learn:
- What parametrically defined functions are and how to differentiate and integrate them: Topics 9.1–9.3.
- What vector-valued functions are and how to differentiate and integrate them: Topics 9.4–9.6.
- How to express functions in a polar coordinate system: Topics 9.7–9.9.
UNIT 10: Infinite Sequences and Series
Exam Weightage: 17–18% ｜ Class periods ~ 17–18
Unit 10 is the last unit of the AP Calculus BC course. In this unit, you’ll learn to understand that a sum of infinitely many terms can converge to a finite value in this unit. You’ll also be investigating graphs, tables, and symbolic expressions for convergent and divergent series and Taylor polynomials.
Big Ideas Incorporated:
- Big Idea 2: Limits – How can the sum of infinitely many discrete terms be a finite value or represent a continuous function?
In this unit, you will learn:
- What an infinite series is and how to find if it converges or diverges: Topics 10.1–10.10.
- What a power series is and how to find its radius and interval of convergence: Topics 10.11–10.13.
- What a Taylor series is and how to use a Taylor polynomial to approximate any function: Topics 10.14–10.15.
Remember to go back and review the big ideas while studying the units. A solid learning process is built on understanding the fundamentals of a subject. With the overview of units, ideas, and concepts discussed above, let's now understand the mathematical practices that you will learn as you move through the course units.
AP Calculus BC Mathematical Practices
As you progress through the course content, you’ll be taught specific skills to approach and solve problems, which are combined under mathematical practices by the College Board. There are four mathematical practices, which are composed of the core skills to help you succeed in the AP Calculus BC exam. The AP course is designed to integrate these practices within the course content so that by the end of the course, you’ll be able to transfer these skills into the AP exam. Now, let’s take a look at these mathematical practices:
Practice 1: Implementing mathematical processes
This is the first mathematical practice you will learn during the AP Calculus BC course. As the name suggests, this mathematical practice will teach you how to solve problems by determining expressions and values by implementing mathematical processes and rules.
Practice 2: Connecting representations
In the second mathematical practice, you'll learn to translate mathematical information from a single representation or across multiple representations by understanding the common underlying structures of mathematical problems.
Practice 3: Justification
The Free-Response Section of the AP Calculus BC exam requires you to justify how you solved a mathematical problem. This mathematical practice will help you develop reasoning skills to establish the steps required to solve problems logically.
Practice 4: Communication and notation
Understanding and resolving a problem are insufficient. You must also understand how to communicate it correctly. You will learn to communicate findings or answers using correct notation, language, and mathematical conventions with the help of this mathematical practice.
Remember to use the core skills and mathematical practices that you learned during your course content. Developing a clear understanding of the concepts and theorems and mastering the ability to apply those concepts effortlessly is the key to achieving a 5 on your AP Calculus BC exam!
Let us help you achieve your dream score! Try our AP Calculus BC practice tests to study smarter and faster!
Frequently Asked Questions
What are the most important topics in AP Calculus BC?
- Unit 10: Infinite Sequences and Series
- Unit 6: Integration and Accumulation of Change
- Unit 9: Parametric Equations, Polar Coordinates, and Vector-Valued Functions
- Unit 5: Analytical Applications of Differentiation
What is the hardest unit topic in AP Calculus BC?
According to the 2023 AP exam results, the most challenging topic in AP Calculus BC was Unit 10: Infinite Sequences and Series.
What AP Calculus BC unit topics aren't in Calculus AB?
The following topics are not included in the AP Calculus AB curriculum:
- Unit 6: Additional techniques of integration
- Unit 7: Euler’s method and logistic models with differential equations
- Unit 8: Arc length and distance traveled along a smooth curve
- Unit 9: Parametric equations, polar coordinates, and vector-valued functions
- Unit 10: Infinite sequences and series
References
- AP Calculus BC. (n.d.). apcentral.collegeboard.org. Retrieved February 16, 2024, from https://apcentral.collegeboard.org/courses/ap-calculus-bc
- AP Calculus AB and BC Course and Exam Description. (2020). apcentral.collegeboard.org. Retrieved February 12, 2024, from https://apcentral.collegeboard.org/media/pdf/ap-calculus-ab-and-bc-course-and-exam-description.pdf
- The 2023 AP Calculus BC scores. (2023, June 30). twitter.com. Retrieved February 28, 2024, from https://twitter.com/AP_Trevor/status/1674514123567005696?lang=en